Difference between revisions of "Graticule"
imported>Jevanyn |
(→Shape) |
||
| (36 intermediate revisions by 21 users not shown) | |||
| Line 1: | Line 1: | ||
A '''graticule''' is a network of geographic lines. We use it to refer to the rectangular{{ref label|1}} zones between the latitude and longitude lines, each 1°×1° in size. | A '''graticule''' is a network of geographic lines. We use it to refer to the rectangular{{ref label|1}} zones between the latitude and longitude lines, each 1°×1° in size. | ||
| + | |||
| + | A graticule may be divided into 100 [[centicule]]s in a 10×10 grid. Thus, centicules are each 0.1°×0.1° in size | ||
| + | |||
== Shape == | == Shape == | ||
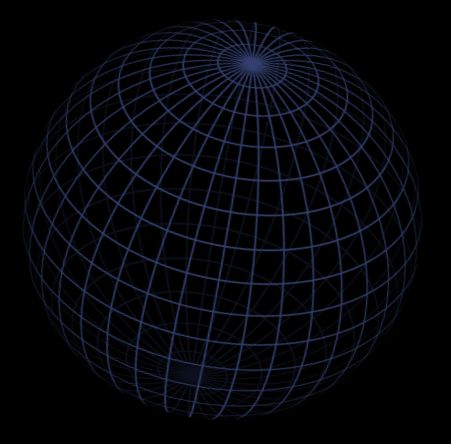
| − | The shape and size of a graticule as measured over the ground (in miles or kilometers) varies with distance from the equator. A graticule near the equator (latitude 0) is almost exactly square shaped (roughly 111×111 km or about 69×69 miles); other graticules are still 111 km in north-south direction, but become narrower and narrower as one goes further away from the equator. For example, the [[ | + | [[File:Graticule.jpg|left|frame|The graticules shown are 10°x10°]] |
| + | The shape and size of a graticule as measured over the ground (in miles or kilometers) varies with distance from the equator. A graticule near the equator (latitude 0) is almost exactly square shaped (roughly 111×111 km or about 69×69 miles); other graticules are still 111 km in north-south direction, but become narrower and narrower as one goes further away from the equator. In other words, the length of one degree of latitude is 111.0 ± 0.2 km, with the last digit uncertain because the Earth isn't a perfect sphere. | ||
| + | |||
| + | One degree of longitude corresponds to (111.0 ± 0.2 km) * cos(L), where L is your latitude. For example, the [[Groningen, Netherlands|Groningen graticule]], at +53° latitude, is 66.8 ± 0.1 km in the east-west direction. Graticules touching on the North and South Poles actually have the shape of a piece of pie, since the polar "border" of such a graticule has length 0. (Most web mapping applications can't display latitudes below -85° or above +85°.) | ||
| + | |||
| + | == Area == | ||
| + | Since the size of each graticule depends only on its latitude, the formula shown below can be used to calculate its area, where R is the radius of the Earth and L is the (absolute value of the) latitude of the graticule (assuming a spherical Earth). | ||
| + | |||
| + | (2πR<sup>2</sup>/360)*(sin(L+1)-sin(L)) | ||
| + | |||
| + | Graticules range in size from 12,400 km<sup>2</sup> at the equator to 108 km<sup>2</sup> at the poles. Thus, the chances of a geohash landing [[Couch Potato Geohash|on your couch]] on any given day increase over 100 times between the two extremes. In fact, in graticule 89, the odds are 1 in 7,200,000 (assuming a 3 m<sup>2</sup> couch with 1 m of navigational error around it), giving you a 50% chance of achieving this goal in less than 10,000 years! | ||
| + | |||
| + | ===More accurate calculation=== | ||
| + | |||
| + | The equatorial circumference of the earth is 40 075.02 km, the meridional circumference is 40 007.86 km. We ignore the minor corrections required by the formulae for ellipsoids. | ||
| + | |||
| + | The height of one graticule is 1/360 of the meridional circumference, or 111.132 94 km. | ||
| + | The width of a graticule varies from bottom to top. (Up is towards the nearest pole for the purposes of this exercise, though mathematically, using either pole will work.) The "base" of the graticule is at the latitude for which the graticule is named; the "top" is at one degree higher. | ||
| + | |||
| + | The length of the base is 1/360 of the equatorial circumference multiplied by the cosine of the latitude: B = 111.319 50 km * cos(L) (L in degrees) | ||
| + | |||
| + | Except at the pole, where the length of the top of the graticule is zero, a graticule is a trapezoid. The area of a trapezoid is 1/2(B1+B2)*H. Thus the area of a graticule whose base is at latitude L is: | ||
| + | |||
| + | A = 12 371.263 314 km<sup>2</sup> * 1/2(cos(L)+cos(L+1)) | ||
| + | |||
| + | Example: [[Santa Cruz, California]], which is at 36, -122. | ||
| + | |||
| + | A = 12 371.263 314 km<sup>2</sup> * 1/2(cos(36)+cos(37)) = 12 371.263 314 km<sup>2</sup> * 0.803 826 = 9 944.346 225 km<sup>2</sup>. | ||
== Numbering == | == Numbering == | ||
| Line 8: | Line 38: | ||
Note that in this numbering 0 is not the same as -0: graticules immediately west of the [[wikipedia:Greenwich meridian|Greenwich meridian]] have the east/west part -0°, and graticules immediately south of the [[wikipedia:Equator|equator]] have the north/south part -0°. For example, graticule (52, 0) is [[Cambridge, United Kingdom]], whereas graticule (52, -0) is the next graticule westwards, [[Northampton, United Kingdom]]. | Note that in this numbering 0 is not the same as -0: graticules immediately west of the [[wikipedia:Greenwich meridian|Greenwich meridian]] have the east/west part -0°, and graticules immediately south of the [[wikipedia:Equator|equator]] have the north/south part -0°. For example, graticule (52, 0) is [[Cambridge, United Kingdom]], whereas graticule (52, -0) is the next graticule westwards, [[Northampton, United Kingdom]]. | ||
| + | |||
| + | There are 360 x 180 = 64,800 graticules on the globe. So far only a fraction of these have been [[All Graticules|named]] and an even smaller fraction actually [[Geohash|geohashed]]. A majority lie in open water. | ||
== Footnotes == | == Footnotes == | ||
| − | {{note label|1|It isn't entirely true that the graticules mark out a rectangular chunk of ground. The side of the graticule closer to the equator will be larger than the one closer to the pole, leading to something more akin to a trapezium. In the limit, at the north and south poles, the graticules become triangular. Add to this the additional complexity of the curvature of the earth and any discussion of the shape of a graticule is either hideously technical or an oversimplification. | + | {{note label|1|It isn't entirely true that the graticules mark out a rectangular chunk of ground. The side of the graticule closer to the equator will be larger than the one closer to the pole, leading to something more akin to a trapezium. In the limit, at the north and south poles, the graticules become triangular. Add to this the additional complexity of the curvature of the earth and any discussion of the shape of a graticule is either hideously technical or an oversimplification. In the context of the [[wikipedia: Web Mercator projection|Mercator projection]] used by OpenStreetMap (the base map for the wiki's various templates) or Google Maps however, the earth is distorted so that the shape is, in fact, rectangular.}} |
| + | |||
| + | [[Category:Definitions]] | ||
| − | [[Category: | + | == See also == |
| + | * [[:Category:All_graticules|All graticules]] | ||
| + | * [[Finding your graticule]] | ||
Latest revision as of 22:37, 19 April 2022
A graticule is a network of geographic lines. We use it to refer to the rectangular[1] zones between the latitude and longitude lines, each 1°×1° in size.
A graticule may be divided into 100 centicules in a 10×10 grid. Thus, centicules are each 0.1°×0.1° in size
Shape
The shape and size of a graticule as measured over the ground (in miles or kilometers) varies with distance from the equator. A graticule near the equator (latitude 0) is almost exactly square shaped (roughly 111×111 km or about 69×69 miles); other graticules are still 111 km in north-south direction, but become narrower and narrower as one goes further away from the equator. In other words, the length of one degree of latitude is 111.0 ± 0.2 km, with the last digit uncertain because the Earth isn't a perfect sphere.
One degree of longitude corresponds to (111.0 ± 0.2 km) * cos(L), where L is your latitude. For example, the Groningen graticule, at +53° latitude, is 66.8 ± 0.1 km in the east-west direction. Graticules touching on the North and South Poles actually have the shape of a piece of pie, since the polar "border" of such a graticule has length 0. (Most web mapping applications can't display latitudes below -85° or above +85°.)
Area
Since the size of each graticule depends only on its latitude, the formula shown below can be used to calculate its area, where R is the radius of the Earth and L is the (absolute value of the) latitude of the graticule (assuming a spherical Earth).
(2πR2/360)*(sin(L+1)-sin(L))
Graticules range in size from 12,400 km2 at the equator to 108 km2 at the poles. Thus, the chances of a geohash landing on your couch on any given day increase over 100 times between the two extremes. In fact, in graticule 89, the odds are 1 in 7,200,000 (assuming a 3 m2 couch with 1 m of navigational error around it), giving you a 50% chance of achieving this goal in less than 10,000 years!
More accurate calculation
The equatorial circumference of the earth is 40 075.02 km, the meridional circumference is 40 007.86 km. We ignore the minor corrections required by the formulae for ellipsoids.
The height of one graticule is 1/360 of the meridional circumference, or 111.132 94 km. The width of a graticule varies from bottom to top. (Up is towards the nearest pole for the purposes of this exercise, though mathematically, using either pole will work.) The "base" of the graticule is at the latitude for which the graticule is named; the "top" is at one degree higher.
The length of the base is 1/360 of the equatorial circumference multiplied by the cosine of the latitude: B = 111.319 50 km * cos(L) (L in degrees)
Except at the pole, where the length of the top of the graticule is zero, a graticule is a trapezoid. The area of a trapezoid is 1/2(B1+B2)*H. Thus the area of a graticule whose base is at latitude L is:
A = 12 371.263 314 km2 * 1/2(cos(L)+cos(L+1))
Example: Santa Cruz, California, which is at 36, -122.
A = 12 371.263 314 km2 * 1/2(cos(36)+cos(37)) = 12 371.263 314 km2 * 0.803 826 = 9 944.346 225 km2.
Numbering
Graticules are numbered with a pair of numbers based on the corner closest to N0°, E0°, so that the graticule a location belongs to can be determined by truncating the degree fraction.
Note that in this numbering 0 is not the same as -0: graticules immediately west of the Greenwich meridian have the east/west part -0°, and graticules immediately south of the equator have the north/south part -0°. For example, graticule (52, 0) is Cambridge, United Kingdom, whereas graticule (52, -0) is the next graticule westwards, Northampton, United Kingdom.
There are 360 x 180 = 64,800 graticules on the globe. So far only a fraction of these have been named and an even smaller fraction actually geohashed. A majority lie in open water.
Footnotes
- ^ It isn't entirely true that the graticules mark out a rectangular chunk of ground. The side of the graticule closer to the equator will be larger than the one closer to the pole, leading to something more akin to a trapezium. In the limit, at the north and south poles, the graticules become triangular. Add to this the additional complexity of the curvature of the earth and any discussion of the shape of a graticule is either hideously technical or an oversimplification. In the context of the Mercator projection used by OpenStreetMap (the base map for the wiki's various templates) or Google Maps however, the earth is distorted so that the shape is, in fact, rectangular.