Difference between revisions of "Talk:2009-06-21 51 -0"
imported>Norsemark (→Post-hash initial thoughts: new section) |
imported>Norsemark (→Claim: new section) |
||
| Line 6: | Line 6: | ||
Gimme some time to pull it together - once it's all there you'll understand why! | Gimme some time to pull it together - once it's all there you'll understand why! | ||
| + | |||
| + | == Claim == | ||
| + | |||
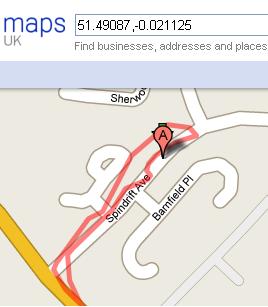
| + | [[Image:2009_06_21_Norse_routex.jpg|thumb|right|Google showing location accurate to 3dp]] | ||
| + | |||
| + | Unless there are any objections, I am going to claim this as a successful attempt. | ||
| + | |||
| + | In spite of technological failure I reached the point to 3 decimal places (which is as detailed at the G1 Application enables) and traced the route along the street wall. The GPS tracker doesn't nail it down to feet and inches and hence shows the dancing route you see here. | ||
| + | |||
| + | Have no problems in calling it a "Blinded By Science" failure if this is insufficient proof, but I'm not sure what else I could do to prove this one. | ||
| + | |||
| + | Any thoughts? | ||
Revision as of 11:48, 22 June 2009
Woah! Just realised how close this is to the meridian. Multihash opportunity?! The two points are only a few miles (and the River Thames) apart. This could be insanely good -- Norsemark 22:45, 19 June 2009 (UTC)
Post-hash initial thoughts
Before I post the report, I'm just going to say that I'm not going to post up any achievements as I'm not convinced they were earned, sadly, but there's a story attached, nonetheless...
Gimme some time to pull it together - once it's all there you'll understand why!
Claim
Unless there are any objections, I am going to claim this as a successful attempt.
In spite of technological failure I reached the point to 3 decimal places (which is as detailed at the G1 Application enables) and traced the route along the street wall. The GPS tracker doesn't nail it down to feet and inches and hence shows the dancing route you see here.
Have no problems in calling it a "Blinded By Science" failure if this is insufficient proof, but I'm not sure what else I could do to prove this one.
Any thoughts?